Loading advertisement...
11-03-2025

CBSE Class 10 Maths Question Paper 2025: Answer Key for All Sets and Detailed Analysis
The CBSE Class 10 Mathematics exam for 2025 has come and gone, leaving students eager to know how well they performed. As always, the exam was designed to test their conceptual understanding, problem-solving skills, and mathematical reasoning. Whether you’re checking for mistakes or calculating your expected score, this blog provides the answer keys for all sets of the CBSE Class 10 Maths paper. Along with the answer keys, we’ll offer a comprehensive analysis of the exam’s difficulty level and tips to help students in the post-exam period.
1. CBSE Class 10 Maths Exam 2025: A Quick Overview
The CBSE Class 10 Mathematics exam follows the prescribed curriculum set by the Central Board of Secondary Education. This year’s paper aimed to assess the students’ proficiency in topics ranging from Algebra, Geometry, and Trigonometry to Statistics and Probability. With a mix of objective and subjective questions, the paper tested students on both their theoretical knowledge and practical problem-solving ability.
The exam was conducted on [date], and since then, students have been eagerly waiting to assess their performance. While the official CBSE answer key will take some time to release, unofficial answer keys for all sets have already been shared by educational experts.
CBSE Class 10 Maths Question Paper 2025 Set 1 2 3 4 PDF Download
We have shared the direct download link for the CBSE Class 10th Maths Question Paper 2025 PDF for all Sets in the table below,
| Set | Link |
|---|---|
| Set 1 | Update soon |
| Set | Update soon |
| Set | Update soon |
| Set | Update soon |
3. Detailed Analysis of the CBSE Class 10 Maths Exam 2025
The exam was designed to balance between theoretical knowledge and practical problem-solving skills. Here’s a breakdown of the paper based on student feedback and expert analysis:
Algebra: The algebra section was relatively straightforward, with questions based on linear equations and quadratic equations. Most students found these problems to be manageable.
Geometry: Geometry had a mix of both easy and challenging questions, particularly in the case of constructions and proofs. Many students found the construction questions a bit tricky but not impossible.
Trigonometry: The Trigonometry questions were a bit more challenging than in previous years. A few students struggled with the trigonometric identities and height and distance problems. However, with proper practice, these could have been tackled well.
Statistics and Probability: This section was considered to be easy to moderate. Students generally performed well on questions related to mean, median, mode, and probability.
CBSE Class 10 Maths Answer Key 2025
Set-1 Answer Key (Maths- STANDARD)
| Question | Answer |
|---|---|
| 1. √0.4 is a/an (A) natural number (B) integer (C) rational number (D) irrational number | (D) irrational number |
| 2. Which of the following cannot be the unit digit of 8n, where n is a natural number? (A) 4 (B) 2 (C) 0 (D) 6 | (C) 0 |
3. Which of the following quadratic equations has real and equal roots? (A) (x+1)2=2x+1 | (B) x2+x=0 |
4. If the zeroes of the polynomial ax2+bx+2a/b are reciprocal of each other, then the value of b is (A) 2 | (B) 1/2 |
| 5. The distance of the point A(-3, -4) from the x-axis is (A) 3 (B) 4 (C) 5 (D) 7 | (B) 4 |
6. In the adjoining figure, PQ || XY || BC, AP = 2 cm, PX = 1.5 cm, and BX = 4 cm. If QY = 0.75 cm, then AQ + CY = 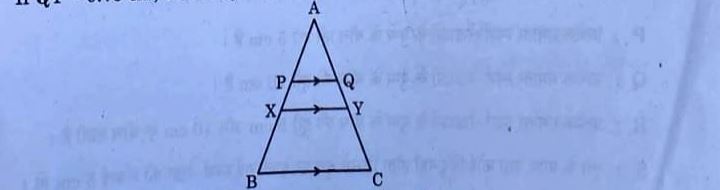
| (B) 4.5 cm |
7.Given △ABC ~ △PQR, ∠A = 30° and ∠Q = 90°. The value of (∠R + ∠B) is: | (C) 150° |
8. Two coins are tossed simultaneously. The probability of getting at least one head is: (A) 1/4 (B) 1/2 (C) 3/4 (D) 1 | (C) 3/4 |
9. In the adjoining figure, PA and PB are tangents to a circle with center O such that ∠P = 90°. If AB = 3√2 cm, then the diameter of the circle is: 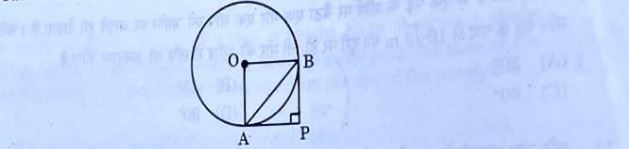 (A) 3√2 cm (B) 6√2 cm (C) 3 cm (D) 6 cm | (D) 6 cm |
10. For a circle with center O and radius 5 cm, which of the following statements is true? P: Distance between every pair of parallel tangents is 5 cm. (A) P | (B) Q |
11. In the adjoining figure, TS is a tangent to a circle with center O. The value of 2x° is: 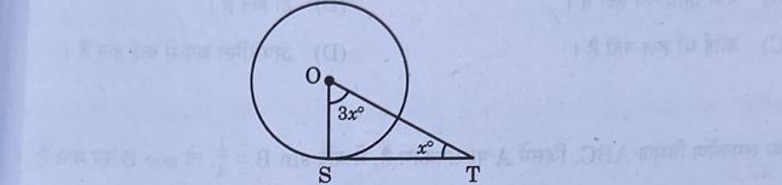 (A) 22.5 | (B) 45 |
12.If y= (2 tan 30°/ 1- tan230°) then x : y = ? Options: | (C) 2: 1 |
13.A peacock sitting on the top of a tree of height 10 m observes a snake moving on the ground. If the snake is 10√3 m away from the base of the tree, then the angle of depression of the snake from the eye of the peacock is: (A) 30° | (C) 30° |
14. If a cone of greatest possible volume is hollowed out from a solid wooden cylinder, then the ratio of the volume of remaining wood to the volume of the cone hollowed out is (A) 1: 1 | (D) 2: 1 |
15.If the mode of some observations is 10 and the sum of mean and median is 25, then the mean and median respectively are: (A) 12 and 13 | (C) 10 and 15 |
16. If the maximum number of students has obtained 52 marks out of 80, then: (A) 52 is the mean of the data. | (C) 52 is the mode of the data. |
17. The system of equations 2x+1=0 and 3y−5=0 has: (A) Unique solution | (A) Unique solution |
18. In a right triangle ABC, right-angled at A, if sinB=1/4, then the value of sec B is: (A) 4 | (D) 4/√15 |
(Assertion-Reason Type) 19. Assertion (A): For any two prime numbers p and q, their HCF is 1 and LCM is p+qp + qp+q. Reason (R): For any two natural numbers, HCF × LCM = product of numbers. | (D) A is false, but R is true. |
20. In an experiment of throwing a die, Assertion (A): Event E₁ (getting a number less than 3) and Event E₂ (getting a number greater than 3) are complementary events. Reason (R): If two events E and F are complementary, then: P(E)+P(F)=1 | (D) A is false, but R is true. |

| Question | Answer |
|---|---|
| 1. The system of equations x+5=0 and 2x−1=0 has (A) No solution (B) Unique solution (C) Two solutions (D) Infinite solutions | (B) Unique solution |
2. In a right triangle ABC, right-angled at A, if sinB=1/4, then the value of secB is | (B) √15/4 |
| 3. √0.4 is a/an (A) natural number (B) integer (C) rational number (D) irrational number | (D) irrational number |
| 4. Which of the following cannot be the unit digit of 8n, where n is a natural number? (A) 4 (B) 2 (C) 0 (D) 6 | (B) 2 |
| 5. Which of the following quadratic equations has real and distinct roots? (A) x2+2x=0 (B) x2+x+1=0 (C) (x−1)2=1−2x (D) 2x2+x+1=0 | (A) x2+2x=0 |
6. If the zeroes of the polynomial ax2+bx+2a/b are reciprocal of each other, then the value of b is (A) 2 | (B) 1/2 |
7. The distance of point (a, -b) from the x-axis is | (C) b |
8. In the adjoining figure, PQ || XY || BC, AP = 2 cm, PX = 1.5 cm, and BX = 4 cm. If QY = 0.75 cm, then AQ + CY = 
| (B) 4.5 cm |
9. Given ΔABC ~ ΔPQR, ∠A = 30° and ∠Q = 90°. The value of (∠R + ∠B) is | (C) 150° |
10. Two coins are tossed simultaneously. The probability of getting at least one head is | (C) 3/4 |
11. In the adjoining figure, PA and PB are tangents to a circle with center O such that ∠P = 90°. If AB = 3√2 cm, then the diameter of the circle is:  (A) 3√2 cm (B) 6√2 cm (C) 3 cm (D) 6 cm | (D) 6 cm |
12. If x = cos 30° - sin 30° and y = tan 60° - cot 60°, then (A) x=y (B) x>у (C) x<у (D) x> 1, y <1 | (C) x<y |
13. For a circle with center O and radius 5 cm, which of the following statements is true? P: Distance between every pair of parallel tangents is 5 cm. (A) P | (B) Q |
14. In the adjoining figure, TS is a tangent to a circle with center O. The value of 2x° is:  (A) 22.5 | (B) 45 |
15. A peacock sitting on the top of a tree of height 10 m observes a snake moving on the ground. If the snake is 10√3 m away from the base of the tree, then the angle of depression of the snake from the eye of the peacock is (A) 30° Answer: (A) 30° | (A) 30° |
16. If a cone of greatest possible volume is hollowed out from a solid wooden cylinder, then the ratio of the volume of remaining wood to the volume of the cone hollowed out is (A) 1:1 (B) 1:3 (C) 2:1 (D) 3:1 | (C) 2:1 |
| Question | Answer |
|---|---|
1. For a circle with centre O and radius 5 cm, which of the following statements is true? P: Distance between every pair of parallel tangents is 5 cm. (A) P | (B) Q |
2. In the adjoining figure, AP and AQ are tangents to the circle with centre O. If reflex ∠POQ = 210°, the value of 2x is (A) 30° | (C) 120° |
3. If x=2 sin60∘ cos60∘and y = sin230° - cos230° and x2= ky2 , the value of k is (A) √3 | (C) 3 |
4. A peacock sitting on the top of a tree of height 10 m observes a snake moving on the ground. If the snake is 10√3 m away from the base of the tree, then the angle of depression of the snake from the eye of the peacock is (A) 30° | (A) 30° |
5. If a cone of greatest possible volume is hollowed out from a solid wooden cylinder, then the ratio of the volume of remaining wood to the volume of the cone hollowed out is (A) 1:1 (B) 1:3 (C) 2:1 (D) 3:1 | (C) 2:1 |
| Question | Answer |
|---|---|
1. In the given figure, the graph of polynomial p(x) is shown. Number of zeroes of p(x) is 
| (A) 3 |
| 2. 22nd term of the A.P: 3, 1/2, −1/2, −3....... is (A) 45/2 (B) -9 (C) −39/2 (D) -21 | (C) −39/2 |
3. The line 2x−3y=62x - 3y = 62x−3y=6 intersects x-axis at (A) (0, -2) | (D) (3,0) |
4. Two identical cones are joined as shown in the figure. If the radius of the base is 4 cm and the slant height of the cone is 6 cm, then the height of the solid is 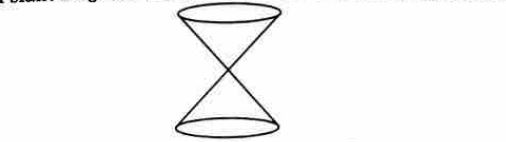 (A) 8 cm | (D) 12 cm |
5. The value of k for which the system of equations 3x−7y=1 and kx+14y=6 is inconsistent, is (A) -6 | (C) 6 |
6. Two dice are rolled together. The probability of getting a sum more than 9 is (A) 5/6 | (B) 5/18 |
7. ABCD is a rectangle with its vertices at (2, -2), (8, 4), (4, 8), and (-2, 2) taken in order. Length of its diagonal is (A) 4√2 | (C) 4√26 |

