CBSE Class 10 Maths Most Repeated Questions For Board Exam 2025

Preparing for the CBSE Class 10 Maths board exam? Practicing the most frequently asked questions can give you an edge. Based on past years' trends, here are the most commonly repeated questions chapter-wise to help you score high.

Chapter-wise Most Repeated Questions

1. Real Numbers

1. Find the HCF & LCM using prime power factorization.
2. Find the HCF & LCM and verify the relationship.
3. Given 2 positive integers a and b expressed as powers of x and y, finding HCF(a, b) or LCM(a, b).
4. Proving a given number is irrational. √2, 3, ... & 2+ 5/3,
5. Word Problem on HCF or LCM.

2. Polynomials

1. Finding the zeroes & the number of zeroes from the given graph.
2. Finding the zeroes of a given quadratic polynomial & verifying the relationship.
3. Finding the quadratic polynomial, given the sum & product of zeroes.
4. If a & ẞ are zeroes of the polynomial, find a polynomial whose zeroes are expressed as various forms of a & ẞ.

3. Pair of Linear Equations in Two Variables

1. Finding whether the given equations are consistent or not.
2. Finding the value of the variable, given that the pair of LE are intersecting, parallel or coincident.
3. Solving the given pair of LE by substitution or Elimination Method. (Specific type: Solve: 99x y + 101 = 499, 101x + 99y = 501)
4. Word Problems: fixed charges, train, age, fraction, reversed digits.

4. Quadratic Equations

1. Finding the nature of roots of the given QE.
2. Given the nature of roots, finding the value of a variable.

Ex: If -3 is a root of the quadratic equation 2x² + px - 15 = 0, while the quadratic equation x² - 4px + k = 0 has equal roots. Find the value of k.

1. Solving QE: simple form or fraction form Ex: 1/x-2 + 2/ x-1 = 6/x: x≠ 0, 1, 2
2. Word problems:

• Speed of flight or boat
• Time taken by taps to fill water

5. Arithmetic Progressions (AP)

1. Finding the nth term from the beginning or end.
2. Finding the common difference of the given AP
3. Finding 'n', for which the nth term of 2 given APs are equal.
4. Case study Qs based on finding the nth term or the sum of n terms

Ex: seats in auditorium, production in nth year, saving in nth year, number of steps covered,...

6. Triangles

1. BPT - Statement & theorem
2. Finding 'x' using BPT
3. Finding 'x' using similarity concept
4. Median related proof question

7. Coordinate Geometry

1. Finding the missing coordinate:

If the point P (6, 2) divides the line segment joining A(6, 5) and B(y, 4) in

Ex: the ratio 3: 1, then the value of y is

1. Applications of Distance Formula - based on types of triangle
2. Applications of Section Formula - based centroid, median, mid point, trisection,
3. Case Study Question based on the above

8. Introduction to Trigonometry & Applications of Trigonometry

1. Given the value of a trigonometric ratio, finding the others.

[Pythagoras Theorem Application]

1. Proof related question based on trigonometric ratios & reciprocals.
2. Questions based on simply applying trigonometric table values.
3. Proof related question based on trigonometric Identities.

9. Circles

1. Finding the angle or height, or length based on the sun's elevation.
2. Height of tower or building.
3. Distance between cars, ships, or two people.
4. Speed related Problem - Car, Bird, flight

10. Constructions

1. Finding the length of a radius chord or tangent.
2. Proof Related questions.
3. Based on the perimeter of the figure circumscribing the circle.

Ex: In the given figure, a circle touches all the four sides of quadrilateral ABCD with AB = 6 cm, BC = 7 cm and CD = 4 cm, then length of AD is

11. Areas Related to Circles

1. Problems related to perimeter or circumference & area of a circle
2. Area of sector (Major & Minor)
3. Area of segment (Major & Minor)
4. Length of arc
5. Related to the ratio of area/perimeter of the circle & the square.

6 Wheel problems

Ex:

→ The diameter of a wheel is 1.26 m. What is the distance covered in 500 revolutions

→ The wheel of a motorcycle is of radius 35 cm. How many revolutions are required to travel a distance of 11 m?

12. Surface Areas and Volumes

1. Problems related to finding the Total Surface Area or Curved Surface Area, or Volume of a combination of the following types of Solids.

13. Statistics and Probability

1. Finding Mean using all 3 methods.
2. Finding Median
3. Finding Mode
4. Finding missing frequencies, given any of the measures of central tendency.
5. Using the empirical relationship to find any of the measures of central tendency, given the other two

14.Probability

1. Coin Related: single, double or triple
2. Dice Related: single or double, prime, odd, even, doublet
3. Deck of Cards Related
4. Complement - (Defective)
5. Bag of colour balls or numbered cards
6. Vowels or Consonants
7. What is the probability that a leap year selected randomly will have 53 Sundays (Or other days)?

CBSE Class 10 Maths Most Repeated Questions

Q1. The King, Queen and Jack of clubs are removed from a pack of 52 cards and then the remaining cards are well shuffled. A card is selected from the remaining cards. Find the probability of getting a card

(i) of spades

(ii) of the black king

(iii) of clubs

(iv) of jacks

(CBSE 2014-2017, 2020)

Q2. In ΔABC, altitude AD and CE intersect each other

other at the point P. Prove that

(1) ΔΑΡΕ ~ ΔCPD

(ii) AP x PD = CP x PE

(iii) ΔADB ~ ΔСЕВ

(iv) AB × CE = BC X AD

(CBSE 2014, 2017, 2020)

Q3. Solve the following pair of linear equations graphically:

x + 3y = 6; 2x-3y = 12

Also, find the area of the triangle by the lines representing the given equation with the y-axis. (CBSE 2012-2020)

Q4. A passenger, while boarding the plane, slipped from the stairs and got hurt. The pilot took the passenger in the emergency clinic at the airport for treatment. Due to this, the plane got delayed by half an hour. To reach the destination 1500 km away in time, so that the passengers could catch the connecting flight, the speed of the plane was increased by 250 km/hour than the usual speed. Find the usual speed of the plane. (CBSE 2011-2020)

Q5. A solid is in the shape of a cone surmounted on a hemisphere, the radius of each of them being 3.5 cm and the total height of sthe olid is 9.5 cm. Find the volume of the solid. [Use π = 22/7] (CBSE 2011-2020)

Q6. A thief runs with a uniform speed of 100 m/minute. After one minute, a policeman runs after the thief to catch him. He goes with a speed of 100 m/minute in the first minute and increases his speed by 10 m/minute every succeeding minute. After how many minutes will the policeman catch the thief? (CBSE 2011, 2012, 2015-2020)

Q7.The median of the following data is 525. Find the values of x and y, if total frequency is 100:(CBSE 2011-2020)

Q8.There are 104 students in class X and 96 students in class IX in a school. In a house examination, the students are to be evenly seated in parallel rows such that no two adjacent rows are of the same class.

(a) Find the maximum number of parallel rows of each class for the seating arrangement.

(b) Also find the number of students of class IX and also of class X in a row (CBSE 2011, 2013, 2015)

Q9. As observed from the top of a 100 m high lighthouse from the sea-level, the angles of depression of two ships are 30° and 45°. If one ship is exactly behind the other on the same side of the lighthouse, find the distance between the two ships. [Use √3 = 1.732] (CBSE 2011-2020)

Q10. Prove that:(1 + cot A+ tan A) (sin A - cos A) = sec³ A-cosec³ A / sec² A. cosec² A

(CBSE 2011-2020)

Q11. Prove that (2+√3)/5 is an irrational number, given that √3 is an irrational number.

Q12.If sides AB, BC and median AD of ΔАВС are proportional to the corresponding sides PQ, QR and median PM of PQR, show that ΔABC ~ ΔPQR.

Q13. Two people are 16 km apart on a straight road. They start walking at the same time. If they walk towards each other with different speeds, they will meet in 2 hours. Had they walked in the same direction with the same speeds as before, they would have met in 8 hours. Find their walking speeds.

Q14. If -4 is a root of the equation x²+2x+4p = 0. Find the value of k for which the quadratic equation x² + px(1+3k) +7(3 + 2k) = 0 has equal roots.

Q15. The ratio of the 11th term to the 18th term of an A.P. is 2: 3. Find the ratio of the 5th term to the 21st term. Also, find the ratio of the sum of the first 5 terms to the sum of the first 21 terms.

Q16. A bird is sitting on the top of an 80 m high tree. From a point on the ground, the angle of elevation of the bird is 45°. The bird flies away horizontally in such a way that it remained at a constant height from the ground. After 2 seconds, the angle of elevation of the bird from the same point is 30°. Find the speed of the flying bird. (Use √3 = 1.732)

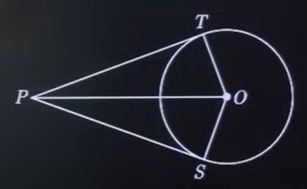
Q17. In the given figure, from a point P, two tangents PT and PS are drawn to a circle with centre O such that <SPT = 120°, Prove that OP = 2PS.

Also check : Maths Mind Map

Tips to Score High in CBSE Class 10 Maths Exam

• Practice previous years' papers to understand question patterns.
• Revise NCERT questions thoroughly as most questions are based on NCERT concepts.
• Understand formulas and theorems clearly and practice their applications.
• Manage time effectively by solving sample papers within the given time limit.
• Use step-wise presentation to get full marks in descriptive questions.

By focusing on these frequently repeated questions and following a strategic preparation plan, you can maximize your score in the CBSE Class 10 Maths Board Exam 2025. Best of luck with your preparation!

Check: